Liuye Notebook
0%

DataStructure-AVL-Tree

发表于 更新于 分类于 ， 阅读次数： Valine：

阅读更多

1 前言

AVL-tree是一种平衡二叉树，搜索的平均复杂度是O(log n)。本篇博客将介绍AVL-tree的两种实现方式，其中第二种方式是参考<STL源码剖析>，较第一种方式更为简单高效，推荐第二种方式

2 定义

在计算机科学中，AVL树是最先发明的自平衡二叉查找树。在AVL树中任何节点的两个子树的高度最大差别为一，所以它也被称为高度平衡树。查找、插入和删除在平均和最坏情况下都是O（log n）。增加和删除可能需要通过一次或多次树旋转来重新平衡这个树

节点的属性

  1. val：节点的值
  2. left：节点的左孩子
  3. right：节点的右孩子
  4. parent：节点的双亲
  5. height：节点的高度
  • 相比于普通的搜索二叉树，AVL树额外维护了一个高度属性，因为AVL树通过该高度属性来判断树的平衡性

平衡性质

  1. 每个节点的左子树与右子树的高度最多不超过1
    • 节点的高度：从给定节点到其最深叶节点所经过的边的数量

3 版本1

3.1 平衡性破坏分析

为了方便描述，定义几个符号

  1. 对于一个节点X，调整(这里的调整特指旋转)之前其高度记为Hx
  2. 仍然对于上述节点X，调整一次后高度记为Hx+

3.1.1 可旋性分析

首先分析左旋，见如下示意图

fig1

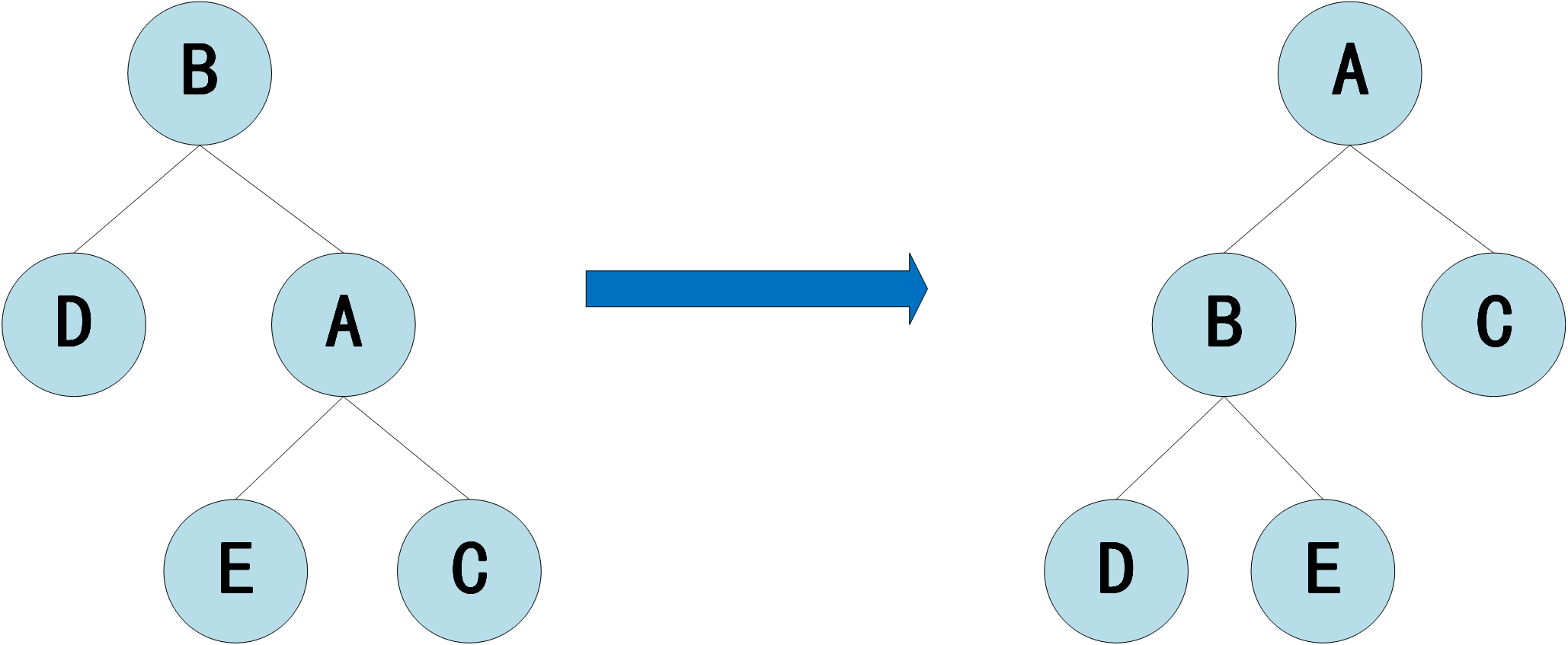
问题1：何时我们会进行左旋操作

只有当右子树的高度大于左子树的高度才会有左旋的需求。以上图为例，即HD=HA-1或HD=HA-2

问题2：何时左旋后能够达到平衡状态

只有HC >= HE时，旋转后该子树的所有节点才满足AVL树的性质

下面对于问题2的结论进行分析

  1. 旋转前，各节点高度如下
    • HD=HA-1或HD=HA-2
    • HC=HA-1
    • HE=HA-1或HE=HA-2
  2. 旋转后，各节点高度如下
    • HD+=HD=HA-1或HA-2
    • HE+=HE=HA-1或HA-2
    • HC+=HC=HA-1
    • 旋转后，B节点平衡，HB+=HA或HB+=HA-1
    • 旋转后，A节点平衡，HA+=HA或HA+=HA+1

然后分析右旋，见如下示意图

fig2

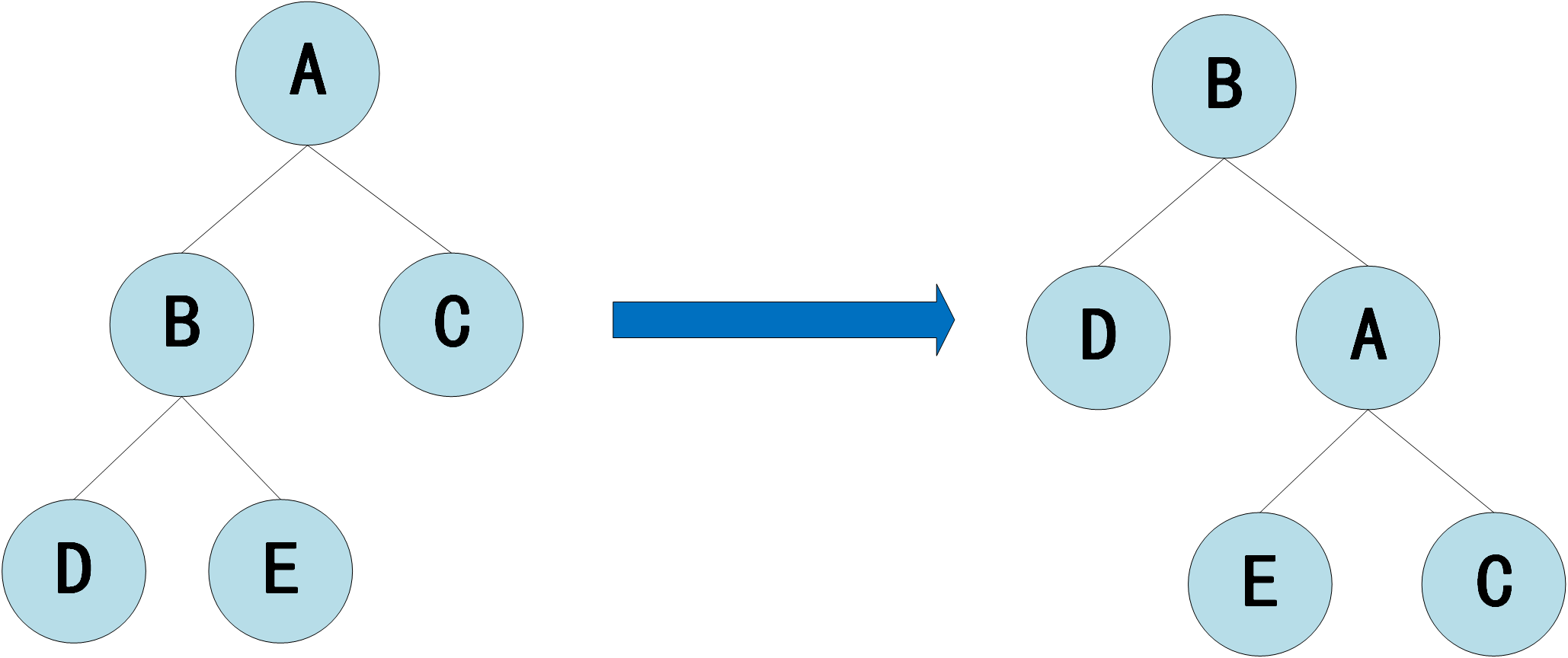
问题1：何时我们会进行右旋操作

只有当左子树的高度大于右子树的高度才会有右旋的需求。以上图为例，即HC=HB-1或HC=HB-2

问题2：何时右旋后能够达到平衡状态

只有HD >= HE时，旋转后该子树的所有节点才满足AVL树的性质

下面对于问题2的结论进行分析

  1. 旋转前，各节点高度如下
    • HC=HB-1或HC=HB-2
    • HD=HB-1
    • HE=HB-1或HE=HB-2
  2. 旋转后，各节点高度如下
    • HD+=HD=HB-1
    • HE+=HE=HB-1或HB-2
    • HC+=HC=HB-1或HB-2
    • 旋转后，A节点平衡，且HA+=HB或HA+=HB-1
    • 旋转后，B节点平衡，且HB+=HB或HB+=HB+1

注意：上述分析仅仅讨论了左旋或者右旋一棵子树时，旋转后该子树是否平衡，但是旋转后该子树的高度是可能变化的，也就是对于该子树的父节点而言，可能又会造成不平衡。因此在AVL性质维护函数中需要充分考虑到这一点

3.1.2 平衡性的维护

当平衡性质被破坏需要右旋来维护性质时

当C为平衡被破坏的节点，且C的左子树比右子树的高度大2，示意图如下

fig3

  • 需要对C进行一次右旋
  • 右旋的前提是HB >= HD
  • 若不满足HB >= HD，则需要首先对A进行一次左旋，而左旋又存在前提
  • 一直往下递归，直至满足旋转条件

其实，以上的过程存在一个可以优化的点：

假设对C节点的右旋条件不满足，即HB < HD，那么此时需要对A节点进行一次左旋。但是A节点左旋后可能会导致A子树的高度发生变化(可能A节点的高度比旋转前少1)。如果A子树的高度少1，那么对于节点C就成为一个平衡节点了，就不需要再进行右旋操作了。但是对于下面将要讲的伪代码中，并没有进行这样的优化。也就意味着不管A子树的高度是否发生变化，C的右旋仍然会执行，这样做并不会破坏平衡性(旋转前后都会处于平衡状态)，只是复杂度增高了

当平衡性质被破坏需要左旋来维护性质时

当A为平衡被破坏的节点，且A的右子树比左子树的高度大2，示意图如下

fig4

  • 需要对A进行一次左旋
  • 左旋的前提是HE >= HD
  • 若不满足HE >= HD，则需要首先对C进行一次右旋，而右旋又存在前提
  • 一直往下递归，直至满足旋转条件

这里存在一个相同的优化点，不再赘述

3.2 伪代码

3.2.1 基本操作

更新指定节点的高度，只有当该节点的左右孩子节点的高度都正确时，才能得到正确的结果

1
2
3
4
AVL-TREE-HEIGHT(T,x)
if x.left.height≥x.right.height   //左右节点均存在
    x.height=x.left.height+1
else x.height=x.right.height+1

将一棵子树替换掉另一棵子树

1
2
3
4
5
6
7
AVL-TREE-TRANSPLANT(T,u,v)   //该函数与红黑树完全一致(都含有哨兵节点)
if u.p==T.nil
    T.root=v
elseif u==u.p.left
    u.p.left=v
else u.p.right=v
    v.p=u.p

3.2.2 左旋和右旋

左旋给定节点，更新旋转后节点的高度，并返回旋转后子树的根节点

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
AVL-TREE-LEFT-ROTATE(T,x)
y=x.right
x.right=y.left
if y.left≠T.nil
    y.left.p=x
y.p=x.p
if x.p== T.nil
    T.root=y
elseif x==x.p.left
    x.p.left=y
else x.p.right=y
y.left=x
x.p=y
AVL-TREE-HEIGHT(T,x)
AVL-TREE-HEIGHT(T,y)
//以上两行顺序不得交换
return y   //返回旋转后的子树根节点

右旋给定节点，更新旋转后节点的高度，并返回旋转后子树的根节点

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
AVL-TREE-RIGH-TROTATE(T,y)
x=y.left
y.left=x.right
if x.right≠T.nil
    x.right.p=y
x.p=y.p
if y.p==T.nil
    root=x
elseif y==y.p.left
    y.p.left=x
else y.p.right=x
x.right=y
y.p=x
AVL-TREE-HEIGHT(T,y)   
AVL-TREE-HEIGHT(T,x)
//以上两行顺序不得交换
return x      //返回旋转后的子树根节点

3.2.3 性质维护

根据指定方向旋转给定节点，旋转后该子树满足平衡的性质，并且输入的节点必须满足如下性质

X节点必定是以X为根节点的子树中唯一不满足平衡性的节点。意味着X节点的孩子子树的所有节点均满足平衡性。因此，必须从下往上找到第一个不平衡的节点来调用该方法

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
AVL-TREE-HOLD-ROTATE(T,x,orientation)
let stack1,stack2 be two stacks//不考虑实际用到的大小，直接用树的大小来分配堆栈空间大小
stack1.push(x)
stack2.push(orientation)
cur=Nil
rotateRoot=Nil //对x尝试旋转后，返回最终旋转后的根节点
curOrientation=INVALID;
while(!stack1.Empty())
    cur=stack1.top()
    curOrientation=stack2.top()
    if curOrientation==LEFT //需要对cur尝试进行左旋
        if cur.right.right.height≥cur.right.left.height
            stack1.pop()
            stack2.pop()
            rotateRoot=AVL-TREE-LEFT-ROTATE(T,cur)
        else
            stack1.push(cur.right)//否则cur右孩子需要尝试进行右旋来调整
            stack2.push(RIGHT);
    elseif curOrientation ==RIGHT//需要对cur尝试进行右旋
        if cur.left.left.height≥cur.left.right.height
            stack1.pop()
            stack2.pop()
            rotateRoot=AVL-TREE-RIGHT-ROTATE(T,cur)
        else
            stack1.push(cur.left) //否则cur左孩子需要尝试进行左旋来调整
            stack2.push(LEFT)
return rotateRoot

3.2.4 插入

插入一个节点

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
AVL-TREE-TREE-INSERT(T,z)
y=T.nil
x=T.root
while x≠T.nil//循环结束时x指向空，y指向上一个x
    y=x
    if z.key<x.key
        x=x.left
    else x=x.right
z.p=y//将这个叶节点作为z的父节点
if y==T.nil
    T.root=z
elseif z.key<y.key
    y.left=z
else y.right=z
z.left=T.nil
z.right=T.nil
AVL-TREE-FIXUP(T,z)

从指定节点向上遍历查找不平衡的节点，并维护平衡树的性质

1
2
3
4
5
6
7
8
9
10
AVL-TREE-FIXUP(T,y) 
if y==T.nil//为了使删除函数也能调用该函数，因为删除函数传入的参数可能是哨兵
    y=y.p
while(y≠T.nil) //沿着y节点向上遍历该条路径
    AVL-TREE-HEIGHT(y)
    if y.left.height==y.right.height+2 //左子树比右子树高2
        y= AVL-TREE-HOLD-ROTATE(T,y,2)
    elseif y.right.height=y.left.height+2    
        y= AVL-TREE-HOLD-ROTATE(T,y,1)
    y=y.p

3.2.5 删除

删除一个节点

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
AVL-TREE-DELETE(T,z)
y=z   //x指向将要移动到y原本位置的节点，或者原本y节点的父节点
if z.left==T.nil
    x=y.right
    AVL-TREE-TRANSPLANT(T,z,z.right)
elseif z.right==T.nil
    x=y.left      
    AVL-TREE-TRANSPLANT(T,z,z.left)
 else y=AVL-TREE-MINIMUM(z.right) //找到z的后继，由于z存在左右孩子，故后继为右子树中的最小值
    x=y.right
    if y.p==z//如果y是z的右孩子，需要将x的parent指向y(使得x为哨兵节点也满足)
        x.p=y
    else AVL-TREE-TRANSPLANT (T,y,y.right)
        y.right=z.right
        y.right.p=y
    AVL-TREE-TRANSPLANT (T,z,y)
    y.left=z.left
    y.left.p=y
AVL-TREE-FIXUP(T,x)

4 版本2

与版本1的实现不同，这个版本的分析将会更加简单，实现效率也会更高

4.1 几类不平衡情况

当插入一个节点后，某节点A为从插入节点往上找到的第一个平衡性被破坏的节点，可以分为如下四种情况，又可分为两大类

  1. 第一类：外侧
    • 插入点位于A的左子节点的左子树–左左
    • 插入点位于A的右子节点的右子树–右右
  2. 第二类：内侧
    • 插入点位于A的左子节点的右子树–左右
    • 插入点位于A的右子节点的左子树–右左

4.1.1 第一类不平衡(以左左为例)

为了方便描述，定义几个符号

  1. 对于一个节点X，调整(这里的调整特指旋转)之前其高度记为Hx
  2. 仍然对于上述节点X，调整一次后高度记为Hx+

插入点位于A的左子节点的左子树–左左，见如下示意图

fig5

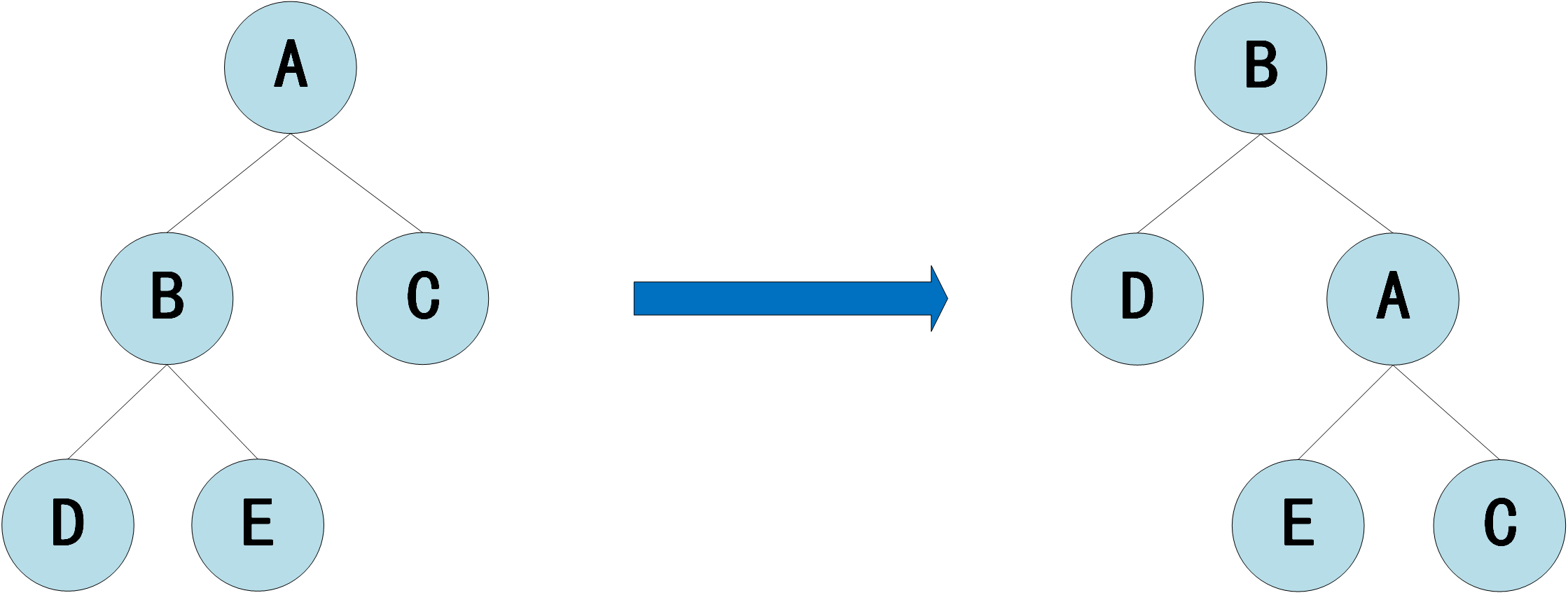
调整前，各节点的高度如下

  • HB=HC+2
  • HA=HC+2(为什么A和B高度相同，因为B的高度已经更新过了，而A仍然是是插入新节点之前的高度，即尚未维护A节点的height字段)
  • HD=HB-1=HC+1
  • HE必定小于HD(否则在新节点插入到节点D为根节点的子树之前，A节点就是不平衡的)，因此HE=HC

右旋调整后，各节点的高度如下

  • HD+=HD=HC+1
  • HE+=HE=HC
  • HC+=HC
  • 由于HE+=HC+，于是A节点平衡，且HA+=HC+1
  • B节点也是平衡的，且HB+=HC+2

可以发现，调整前后子树根节点的高度都是HC+2，因此该节点上层的节点的平衡性不会被破坏，于是通过一次右旋，不平衡性即被消除

4.1.2 第二类不平衡(以左右为例)

为了方便描述，定义几个符号

  1. 对于一个节点X，调整(这里的调整特指旋转)之前其高度记为Hx
  2. 仍然对于上述节点X，调整一次后高度记为Hx+
  3. 仍然对于上述节点X，调整二次后高度记为Hx++

插入点位于A的左子节点的右子树–左右，见如下示意图

fig6

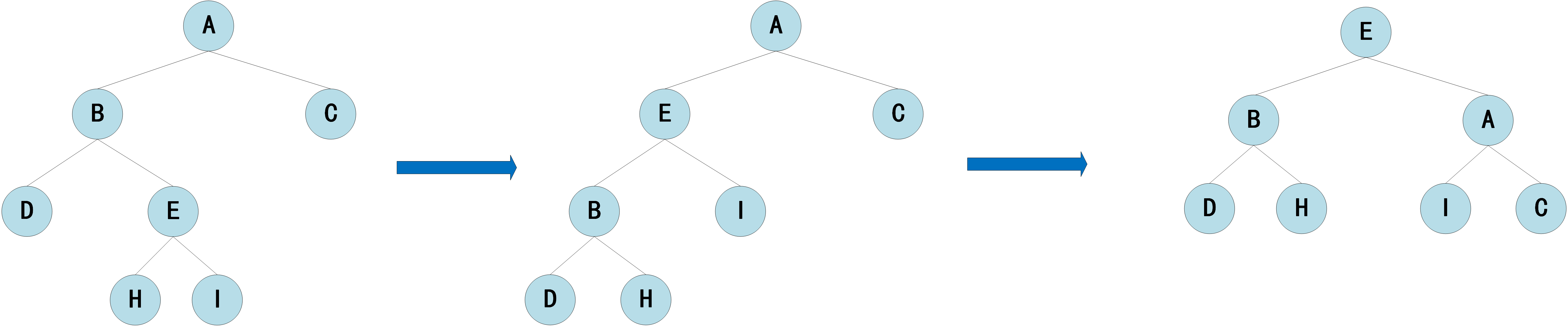
调整前，各节点的高度如下

  • HB=HC+2
  • HA=HC+2(为什么A和B高度相同，因为B的高度已经更新过了，而A仍然是是插入新节点之前的高度，即尚未维护A节点的height字段)
  • HE=HB-1=HC+1
  • HD必定小于HE，因此HD=HC
  • HH与HI至少有一个是HC，另一个可以是HC或HC-1

对B节点进行一次左旋后，各节点高度如下

  • HD+=HD=HC
  • HH+=HH=HC or HC-1
  • HI+=HI=HC or HC-1
  • HC+=HC
  • HA+=HA=HC+2
  • HB+=HC+1
  • 当HI+=HC-1时，节点E可能是不平衡的，但是没关系，这只是个中间状态，HE=HC+2

对A节点进行一次右旋，各节点高度如下

  • HD++=HD+=HC
  • HH++=HH+= HC or HC-1
  • HI++=HI+=HC or HC-1
  • HC++=HC+=HC
  • 旋转后，B节点平衡，HB++=HC+1
  • 旋转后，A节点平衡，HA++=HC+1
  • 因此旋转后，E节点平衡，HE++=HC+2

可以发现，调整前后子树根节点的高度都是HC+2，因此该节点上层的节点的平衡性不会被破坏，于是通过一次左旋和一次右旋，不平衡性即被消除

4.2 伪代码

4.2.1 基本操作

更新指定节点的高度，只有当该节点的左右孩子节点的高度都正确时，才能得到正确的结果

1
2
3
4
AVL-TREE-HEIGHT(T,x)
if x.left.height≥x.right.height   //左右节点均存在
    x.height=x.left.height+1
else x.height=x.right.height+1

将一棵子树替换掉另一棵子树

1
2
3
4
5
6
7
AVL-TREE-TRANSPLANT(T,u,v)   //该函数与红黑树完全一致(都含有哨兵节点)
if u.p==T.nil
    T.root=v
elseif u==u.p.left
    u.p.left=v
else u.p.right=v
    v.p=u.p

4.2.2 左旋和右旋

左旋给定节点，更新旋转后节点的高度，并返回旋转后子树的根节点

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
AVL-TREE-LEFT-ROTATE(T,x)
y=x.right
x.right=y.left
if y.left≠T.nil
    y.left.p=x
y.p=x.p
if x.p== T.nil
    T.root=y
elseif x==x.p.left
    x.p.left=y
else x.p.right=y
y.left=x
x.p=y
AVL-TREE-HEIGHT(T,x)
AVL-TREE-HEIGHT(T,y)
//以上两行顺序不得交换
return y   //返回旋转后的子树根节点

右旋给定节点，更新旋转后节点的高度，并返回旋转后子树的根节点

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
AVL-TREE-RIGHT-ROTATE(T,y)
x=y.left
y.left=x.right
if x.right≠T.nil
    x.right.p=y
x.p=y.p
if y.p==T.nil
    root=x
elseif y==y.p.left
    y.p.left=x
else y.p.right=x
x.right=y
y.p=x
AVL-TREE-HEIGHT(T,y)   
AVL-TREE-HEIGHT(T,x)
//以上两行顺序不得交换
return x      //返回旋转后的子树根节点

4.2.3 插入

插入一个节点

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
AVL-TREE-INSERT(T,z)
y=T.nil
x=T.root
while x≠T.nil//循环结束时x指向空，y指向上一个x
    y=x
    if z.key<x.key
        x=x.left
    else x=x.right
z.p=y//将这个叶节点作为z的父节点
if y==T.nil
    T.root=z
elseif z.key<y.key
    y.left=z
else y.right=z
z.left=T.nil
z.right=T.nil
AVL-TREE-BALANCE-FIX (T,z)

维护平衡性质，分别讨论第一类第二类的四种不平衡情况

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
AVL-TREE--BALANCE-FIX(T,z)
originHigh=z.h
AVL-TREE-HEIGHT(z)
r=z
if z.left.h==z.right.h+2
    if z.left.left.h>=z.left.right.h   //第一类，等号在插入过程中不可能取到，删除过程中能取到
        r=AVL-TREE-RIGHT-ROTATE(z)
    elseif z.left.left.h<z.left.right.h     //第二类
        AVL-TREE-LEFT-ROTATE(z.left)
        r=AVL-TREE-RIGHT-ROTATE(z)
    //不可能出现左右子树高度相同的情况，但是DELETE-FIX中可能出现，注意
elseif z.right.h==z.left.h+2
    if z.right.right.h>=z.right.left.h   //第一类，等号在插入过程中不可能取到，删除过程中能取到
        r=AVL-TREE-LEFT-ROTATE(z)
    elseif z.right.right.h<z.right.left.h      //第二类
        AVL-TREE-RIGHT-ROTATE(z.right)
        r=AVL-TREE-LEFT-ROTATE(z)
    //不可能出现左右子树高度相同的情况，但是DELETE-FIX中可能出现，注意
if r.h!=originHigh and r!=root
    AVL-TREE--BALANCE-FIX(r.parent)

4.2.4 删除

删除给定关键字

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
AVL-TREE-DELETE(T,z)
y=z   //x指向将要移动到y原本位置的节点，或者原本y节点的父节点
p=y.parent   //p为被删除节点的父节点
if z.left==T.nil
    AVL-TREE-TRANSPLANT(T,z,z.right)
elseif z.right==T.nil
    AVL-TREE-TRANSPLANT(T,z,z.left)
else y=AVL-TREE-MINIMUM(z.right) //找到z的后继，由于z存在左右孩子，故后继为右子树中的最小值
    if y==z.right    //这个边界判断必须，因为p必须定位到被删除节点的父节点
        p=y
    else
        p=y.parent
    AVL-TREE-TRANSPLANT(y,y.right)
    y.right=z.right
    y.right.parent=y
    y.left=z.left
    y.left.parent=y
    AVL-TREE-TRANSPLANT (T,z,y)
    y.height=z.height
if p!=nil
    AVL-TREE--BALANCE-FIX(p)

5 Java源码

5.1 节点定义

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
public class AVLTreeNode {
    /**
     * 该节点的高度(从该节点到叶节点的最多边数)
     */
    int h;

    /**
     * 节点的值
     */
    int val;

    /**
     * 该节点的左孩子节点，右孩子节点，父节点
     */
    AVLTreeNode left, right, parent;

    public AVLTreeNode(int val) {
        h = 0;
        this.val = val;
        left = null;
        right = null;
        parent = null;
    }
}

5.2 版本1

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
100
101
102
103
104
105
106
107
108
109
110
111
112
113
114
115
116
117
118
119
120
121
122
123
124
125
126
127
128
129
130
131
132
133
134
135
136
137
138
139
140
141
142
143
144
145
146
147
148
149
150
151
152
153
154
155
156
157
158
159
160
161
162
163
164
165
166
167
168
169
170
171
172
173
174
175
176
177
178
179
180
181
182
183
184
185
186
187
188
189
190
191
192
193
194
195
196
197
198
199
200
201
202
203
204
205
206
207
208
209
210
211
212
213
214
215
216
217
218
219
220
221
222
223
224
225
226
227
228
229
230
231
232
233
234
235
236
237
238
239
240
241
242
243
244
245
246
247
248
249
250
251
252
253
254
255
256
257
258
259
260
261
262
263
264
265
266
267
268
269
270
271
272
273
274
275
276
277
278
279
280
281
282
283
284
285
286
287
288
289
290
291
292
293
294
295
296
297
298
299
300
301
302
303
304
305
306
307
308
309
310
311
312
313
314
315
316
317
318
319
320
321
322
323
324
325
326
327
328
329
330
331
332
333
334
335
336
337
338
339
340
341
342
343
344
345
346
package org.liuyehcf.algorithm.datastructure.tree.avltree;

import java.util.*;

/**
 * Created by Liuye on 2017/4/27.
 */

public class AVLTree1 {
    private enum RotateOrientation {
        INVALID,
        LEFT,
        RIGHT
    }

    private AVLTreeNode root;

    private AVLTreeNode nil;
    private Map<AVLTreeNode, Integer> highMap;

    public AVLTree1() {
        nil = new AVLTreeNode(0);
        nil.left = nil;
        nil.right = nil;
        nil.parent = nil;
        root = nil;
    }

    public void insert(int val) {
        AVLTreeNode x = root;
        AVLTreeNode y = nil;
        AVLTreeNode z = new AVLTreeNode(val);
        while (x != nil) {
            y = x;
            if (val < x.val) {
                x = x.left;
            } else {
                x = x.right;
            }
        }
        z.parent = y;
        if (y == nil) {
            root = z;
        } else if (z.val < y.val) {
            y.left = z;
        } else {
            y.right = z;
        }
        z.left = nil;
        z.right = nil;
        fixUp(z);
        if (!check())
            throw new RuntimeException();
    }

    private void fixUp(AVLTreeNode y) {
        if (y == nil) {
            y = y.parent;
        }
        while (y != nil) {
            updateHigh(y);
            if (y.left.h == y.right.h + 2)
                y = holdRotate(y, RotateOrientation.RIGHT);
            else if (y.right.h == y.left.h + 2)
                y = holdRotate(y, RotateOrientation.LEFT);
            y = y.parent;
        }
    }

    private AVLTreeNode holdRotate(AVLTreeNode x, RotateOrientation orientation) {
        LinkedList<AVLTreeNode> stack1 = new LinkedList<AVLTreeNode>();
        LinkedList<RotateOrientation> stack2 = new LinkedList<RotateOrientation>();
        stack1.push(x);
        stack2.push(orientation);
        AVLTreeNode cur = nil;
        AVLTreeNode rotateRoot = nil;
        RotateOrientation curOrientation = RotateOrientation.INVALID;
        while (!stack1.isEmpty()) {
            cur = stack1.peek();
            curOrientation = stack2.peek();
            if (curOrientation == RotateOrientation.LEFT) {
                if (cur.right.right.h >= cur.right.left.h) {
                    stack1.pop();
                    stack2.pop();
                    rotateRoot = leftRotate(cur);
                } else {
                    stack1.push(cur.right);
                    stack2.push(RotateOrientation.RIGHT);
                }
            } else if (curOrientation == RotateOrientation.RIGHT) {
                if (cur.left.left.h >= cur.left.right.h) {
                    stack1.pop();
                    stack2.pop();
                    rotateRoot = rightRotate(cur);
                } else {
                    stack1.push(cur.left);
                    stack2.push(RotateOrientation.LEFT);
                }
            }
        }
        return rotateRoot;
    }

    private void updateHigh(AVLTreeNode z) {
        z.h = Math.max(z.left.h, z.right.h) + 1;
    }

    /**
     * 左旋
     *
     * @param x
     * @return 返回旋转后的根节点
     */
    private AVLTreeNode leftRotate(AVLTreeNode x) {
        AVLTreeNode y = x.right;
        x.right = y.left;
        if (y.left != nil) {
            y.left.parent = x;
        }
        y.parent = x.parent;
        if (x.parent == nil) {
            root = y;
        } else if (x == x.parent.left) {
            x.parent.left = y;
        } else {
            x.parent.right = y;
        }
        y.left = x;
        x.parent = y;

        //更新高度
        updateHigh(x);
        updateHigh(y);
        return y;
    }

    /**
     * 右旋
     *
     * @param y
     * @return 返回旋转后的根节点
     */
    private AVLTreeNode rightRotate(AVLTreeNode y) {
        AVLTreeNode x = y.left;
        y.left = x.right;
        if (x.right != nil) {
            x.right.parent = y;
        }
        x.parent = y.parent;
        if (y.parent == nil) {
            root = x;
        } else if (y == y.parent.left) {
            y.parent.left = x;
        } else {
            y.parent.right = x;
        }
        x.right = y;
        y.parent = x;

        //更新高度
        updateHigh(y);
        updateHigh(x);
        return x;
    }

    private boolean check() {
        highMap = new HashMap<AVLTreeNode, Integer>();
        return checkHigh(root) && checkBalance(root);
    }

    private boolean checkHigh(AVLTreeNode root) {
        if (root == nil) return true;
        return checkHigh(root.left) && checkHigh(root.right) && root.h == high(root);
    }

    private int high(AVLTreeNode root) {
        if (root == nil) {
            return 0;
        }
        if (highMap.containsKey(root)) return highMap.get(root);
        int leftHigh = high(root.left);
        int rightHigh = high(root.right);
        highMap.put(root, Math.max(leftHigh, rightHigh) + 1);
        return highMap.get(root);
    }

    private boolean checkBalance(AVLTreeNode root) {
        if (root == nil) {
            return true;
        }
        int leftHigh = root.left.h;
        int rightHigh = root.right.h;
        if (Math.abs(leftHigh - rightHigh) == 2) return false;
        return checkBalance(root.left) && checkBalance(root.right);
    }

    public boolean search(int val) {
        return search(root, val) != nil;
    }

    private AVLTreeNode search(AVLTreeNode x, int val) {
        while (x != nil) {
            if (x.val == val) return x;
            else if (val < x.val) {
                x = x.left;
            } else {
                x = x.right;
            }
        }
        return nil;
    }

    public void delete(int val) {
        AVLTreeNode z = search(root, val);
        if (z == nil) {
            throw new RuntimeException();
        }
        AVLTreeNode y = z;
        AVLTreeNode x = nil;
        if (z.left == nil) {
            x = y.right;
            transplant(z, z.right);
        } else if (z.right == nil) {
            x = y.left;
            transplant(z, z.left);
        } else {
            y = min(z.right);
            x = y.right;
            if (y.parent == z) {
                x.parent = y;
            } else {
                transplant(y, y.right);
                y.right = z.right;
                y.right.parent = y;
            }
            transplant(z, y);

            y.left = z.left;
            y.left.parent = y;

            //todo 这里不需要更新p的高度,因为p的子树的高度此时并不知道是否正确,因此更新也没有意义,这也是deleteFixBalance必须遍历到root的原因
        }
        fixUp(x);
        if (!check())
            throw new RuntimeException();
    }

    private void transplant(AVLTreeNode u, AVLTreeNode v) {
        v.parent = u.parent;
        if (u.parent == nil) {
            root = v;
        } else if (u == u.parent.left) {
            u.parent.left = v;
        } else {
            u.parent.right = v;
        }
    }

    private AVLTreeNode min(AVLTreeNode x) {
        while (x.left != nil) {
            x = x.left;
        }
        return x;
    }

    public void inOrderTraverse() {
        inOrderTraverse(root);
        System.out.println();
    }

    public void levelOrderTraversal() {
        Queue<AVLTreeNode> queue = new LinkedList<AVLTreeNode>();
        queue.offer(root);
        while (!queue.isEmpty()) {
            int len = queue.size();
            for (int i = 0; i < len; i++) {
                AVLTreeNode peek = queue.poll();
                System.out.print("[" + peek.val + "," + peek.h + "], ");
                if (peek.left != nil) queue.offer(peek.left);
                if (peek.right != nil) queue.offer(peek.right);
            }
        }
        System.out.println();
    }

    private void inOrderTraverse(AVLTreeNode root) {
        if (root != nil) {
            inOrderTraverse(root.left);
            System.out.print(root.val + ", ");
            inOrderTraverse(root.right);
        }
    }

    public static void main(String[] args) {
        long start = System.currentTimeMillis();

        Random random = new Random();

        int TIMES = 10;

        while (--TIMES > 0) {
            System.out.println("剩余测试次数: " + TIMES);
            AVLTree1 avlTree2 = new AVLTree1();

            int N = 1000;
            int M = N / 2;

            Set<Integer> set = new HashSet<Integer>();
            for (int i = 0; i < N; i++) {
                set.add(random.nextInt());
            }

            List<Integer> list = new ArrayList<Integer>(set);
            Collections.shuffle(list, random);
            //插入N个数据
            for (int i : list) {
                avlTree2.insert(i);
            }

            //删除M个数据
            Collections.shuffle(list, random);

            for (int i = 0; i < M; i++) {
                set.remove(list.get(i));
                avlTree2.delete(list.get(i));
            }

            //再插入M个数据
            for (int i = 0; i < M; i++) {
                int k = random.nextInt();
                set.add(k);
                avlTree2.insert(k);
            }
            list.clear();
            list.addAll(set);
            Collections.shuffle(list, random);

            //再删除所有元素
            for (int i : list) {
                avlTree2.delete(i);
            }
        }
        long end = System.currentTimeMillis();
        System.out.println("Run time: " + (end - start) / 1000 + "s");
    }
}

5.3 版本2

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
100
101
102
103
104
105
106
107
108
109
110
111
112
113
114
115
116
117
118
119
120
121
122
123
124
125
126
127
128
129
130
131
132
133
134
135
136
137
138
139
140
141
142
143
144
145
146
147
148
149
150
151
152
153
154
155
156
157
158
159
160
161
162
163
164
165
166
167
168
169
170
171
172
173
174
175
176
177
178
179
180
181
182
183
184
185
186
187
188
189
190
191
192
193
194
195
196
197
198
199
200
201
202
203
204
205
206
207
208
209
210
211
212
213
214
215
216
217
218
219
220
221
222
223
224
225
226
227
228
229
230
231
232
233
234
235
236
237
238
239
240
241
242
243
244
245
246
247
248
249
250
251
252
253
254
255
256
257
258
259
260
261
262
263
264
265
266
267
268
269
270
271
272
273
274
275
276
277
278
279
280
281
282
283
284
285
286
287
288
289
290
291
292
293
294
295
296
297
298
299
300
301
302
303
304
305
306
307
308
309
310
311
312
313
314
315
316
317
318
319
320
321
322
323
324
325
326
327
328
package org.liuyehcf.algorithm.datastructure.tree.avltree;

import java.util.*;

/**
 * Created by liuye on 2017/4/24 0024.
 */

public class AVLTree2 {
    private AVLTreeNode root;

    private AVLTreeNode nil;
    private Map<AVLTreeNode, Integer> highMap;

    public AVLTree2() {
        nil = new AVLTreeNode(0);
        nil.left = nil;
        nil.right = nil;
        nil.parent = nil;
        root = nil;
    }

    public void insert(int val) {
        AVLTreeNode x = root;
        AVLTreeNode y = nil;
        AVLTreeNode z = new AVLTreeNode(val);
        while (x != nil) {
            y = x;
            if (val < x.val) {
                x = x.left;
            } else {
                x = x.right;
            }
        }
        z.parent = y;
        if (y == nil) {
            root = z;
        } else if (z.val < y.val) {
            y.left = z;
        } else {
            y.right = z;
        }
        z.left = nil;
        z.right = nil;
        balanceFix(z);
        if (!check())
            throw new RuntimeException();
    }

    private void balanceFix(AVLTreeNode z) {
        //当前节点的初始高度
        int originHigh = z.h;

        updateHigh(z);

        //经过调整后的子树根节点(调整之前子树根节点为z)
        AVLTreeNode r = z;

        if (z.left.h == z.right.h + 2) {
            //todo 这里的等号非常重要(插入过程时不可能取等号，删除过程可能取等号)
            if (z.left.left.h >= z.left.right.h) {
                r = rightRotate(z);
            } else if (z.left.left.h < z.left.right.h) {
                leftRotate(z.left);
                r = rightRotate(z);
            }

        } else if (z.right.h == z.left.h + 2) {
            //todo 这里的等号非常重要(插入过程时不可能取等号，删除过程可能取等号)
            if (z.right.right.h >= z.right.left.h) {
                r = leftRotate(z);
            } else if (z.right.right.h < z.right.left.h) {
                rightRotate(z.right);
                r = leftRotate(z);
            }
        }

        //递归其父节点
        if (r.h != originHigh && r != root)
            balanceFix(r.parent);
    }

    private void updateHigh(AVLTreeNode z) {
        z.h = Math.max(z.left.h, z.right.h) + 1;
    }

    /**
     * 左旋
     *
     * @param x
     * @return 返回旋转后的根节点
     */
    private AVLTreeNode leftRotate(AVLTreeNode x) {
        AVLTreeNode y = x.right;
        x.right = y.left;
        if (y.left != nil) {
            y.left.parent = x;
        }
        y.parent = x.parent;
        if (x.parent == nil) {
            root = y;
        } else if (x == x.parent.left) {
            x.parent.left = y;
        } else {
            x.parent.right = y;
        }
        y.left = x;
        x.parent = y;

        //更新高度
        updateHigh(x);
        updateHigh(y);
        return y;
    }

    /**
     * 右旋
     *
     * @param y
     * @return 返回旋转后的根节点
     */
    private AVLTreeNode rightRotate(AVLTreeNode y) {
        AVLTreeNode x = y.left;
        y.left = x.right;
        if (x.right != nil) {
            x.right.parent = y;
        }
        x.parent = y.parent;
        if (y.parent == nil) {
            root = x;
        } else if (y == y.parent.left) {
            y.parent.left = x;
        } else {
            y.parent.right = x;
        }
        x.right = y;
        y.parent = x;

        //更新高度
        updateHigh(y);
        updateHigh(x);
        return x;
    }

    private boolean check() {
        highMap = new HashMap<AVLTreeNode, Integer>();
        return checkHigh(root) && checkBalance(root);
    }

    private boolean checkHigh(AVLTreeNode root) {
        if (root == nil) return true;
        return checkHigh(root.left) && checkHigh(root.right) && root.h == high(root);
    }

    private int high(AVLTreeNode root) {
        if (root == nil) {
            return 0;
        }
        if (highMap.containsKey(root)) return highMap.get(root);
        int leftHigh = high(root.left);
        int rightHigh = high(root.right);
        highMap.put(root, Math.max(leftHigh, rightHigh) + 1);
        return highMap.get(root);
    }

    private boolean checkBalance(AVLTreeNode root) {
        if (root == nil) {
            return true;
        }
        int leftHigh = root.left.h;
        int rightHigh = root.right.h;
        if (Math.abs(leftHigh - rightHigh) == 2) return false;
        return checkBalance(root.left) && checkBalance(root.right);
    }

    public boolean search(int val) {
        return search(root, val) != nil;
    }

    private AVLTreeNode search(AVLTreeNode x, int val) {
        while (x != nil) {
            if (x.val == val) return x;
            else if (val < x.val) {
                x = x.left;
            } else {
                x = x.right;
            }
        }
        return nil;
    }

    public void delete(int val) {
        AVLTreeNode z = search(root, val);
        if (z == nil) {
            throw new RuntimeException();
        }
        //y代表真正被删除的节点
        AVLTreeNode y = z;
        //x为被删除节点的父节点，如果平衡被破坏，从该节点开始
        AVLTreeNode p = y.parent;
        if (z.left == nil) {
            transplant(z, z.right);
        } else if (z.right == nil) {
            transplant(z, z.left);
        } else {
            y = min(z.right);
            //todo 这里的分类讨论非常重要,否则将会定位到错误的父节点
            if (y == z.right) {
                p = y;
            } else {
                p = y.parent;
            }
            transplant(y, y.right);

            //todo 下面六句可以用z.val=y.val来代替,效果一样
            y.right = z.right;
            y.right.parent = y;

            y.left = z.left;
            y.left.parent = y;

            transplant(z, y);
            y.h = z.h;//todo 这里高度必须维护
            //todo 这里不需要更新p的高度,因为p的子树的高度此时并不知道是否正确,因此更新也没有意义,这也是deleteFixBalance必须遍历到root的原因
        }
        if (p != nil)
            balanceFix(p);
        if (!check())
            throw new RuntimeException();
    }

    private void transplant(AVLTreeNode u, AVLTreeNode v) {
        v.parent = u.parent;
        if (u.parent == nil) {
            root = v;
        } else if (u == u.parent.left) {
            u.parent.left = v;
        } else {
            u.parent.right = v;
        }
    }

    private AVLTreeNode min(AVLTreeNode x) {
        while (x.left != nil) {
            x = x.left;
        }
        return x;
    }

    public void inOrderTraverse() {
        inOrderTraverse(root);
        System.out.println();
    }

    public void levelOrderTraversal() {
        Queue<AVLTreeNode> queue = new LinkedList<AVLTreeNode>();
        queue.offer(root);
        while (!queue.isEmpty()) {
            int len = queue.size();
            for (int i = 0; i < len; i++) {
                AVLTreeNode peek = queue.poll();
                System.out.print("[" + peek.val + "," + peek.h + "], ");
                if (peek.left != nil) queue.offer(peek.left);
                if (peek.right != nil) queue.offer(peek.right);
            }
        }
        System.out.println();
    }

    private void inOrderTraverse(AVLTreeNode root) {
        if (root != nil) {
            inOrderTraverse(root.left);
            System.out.print(root.val + ", ");
            inOrderTraverse(root.right);
        }
    }

    public static void main(String[] args) {
        long start = System.currentTimeMillis();

        Random random = new Random();

        int TIMES = 10;

        while (--TIMES > 0) {
            System.out.println("剩余测试次数: " + TIMES);
            AVLTree2 avlTree = new AVLTree2();

            int N = 10000;
            int M = N / 2;

            List<Integer> list = new ArrayList<Integer>();
            for (int i = 0; i < N; i++) {
                list.add(random.nextInt());
            }

            Collections.shuffle(list, random);
            //插入N个数据
            for (int i : list) {
                avlTree.insert(i);
            }

            //删除M个数据
            Collections.shuffle(list, random);

            for (int i = 0; i < M; i++) {
                int k = list.get(list.size() - 1);
                list.remove(list.size() - 1);
                avlTree.delete(k);
            }

            //再插入M个数据
            for (int i = 0; i < M; i++) {
                int k = random.nextInt();
                list.add(k);
                avlTree.insert(k);
            }
            Collections.shuffle(list, random);

            //再删除所有元素
            for (int i : list) {
                avlTree.delete(i);
            }
        }
        long end = System.currentTimeMillis();
        System.out.println("Run time: " + (end - start) / 1000 + "s");
    }
}